| sign | Meaning of the sign | |
|---|---|---|
| d | wire diameter(φ) |
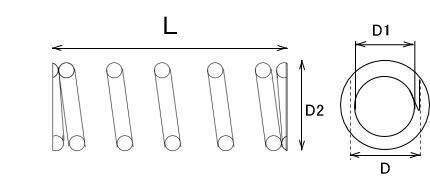  |
| D1 | inside diameter of coil (㎜) | |
| D2 | outside diameter of coil(㎜) | |
| D | mean diameter of coil (D1+D2)/2 |
|
| Na | number of active coils | |
| Nt | total number of turns | |
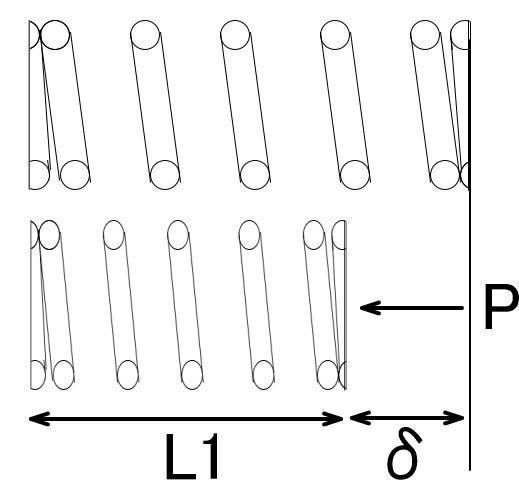
| L | free length(㎜) | |
| P | load(N) | |
| δ | spring travel(㎜) | |
| k | spring constant(N/㎜) | |
| G | modulus of transverse elasticity(N/㎟) | |
| c | spring index(D/d) |
| material | modulus of transverse elasticity(N/㎜) |
| hard steel wire | 78500 |
| piano wire | 78500 |
| oil tempered wires | 78500 |
| stainless steel | 68500 |
Calculate the weight of the spring
Sample⇒piano wireφ2.0 active coils 5 coil diameter φ15.0
1.Find the Mass of the spring
cross section × The length of the spring = Mass of the spring
formula ⇒ (1.0×1.0×3.14)×(15.0×3.14×7)=3.14×329.7=1035.258㎣
2.Find the weight of the spring
mass × specific gravity = weight of the spring
formula ⇒ 1035.258㎣ × 0.00784g/㎥ = 8.116g
Calculate the fixed number of the spring
Sample⇒piano wireφ2.0 active coils5 coil diameteφ15.0
spring constant = (modulus of transverse elasticity×wire diameter to the 4)÷(8×number of active coils×mean diameter to the 3)
formula ⇒ (78,500×2.0^4)÷(8×5×15.0^3)=9.304N/㎜
Calculate the load
Spring properties mentioned above. free length 30㎜ Installation length 25㎜.
load = spring travel × spring constant │ spring travel = free length - installation length
formula ⇒ (30-25)×9.304 = 46.52N
Calculate the stress of the spring
When assume it spring properties mentioned above.
spring index(c)=mean diameter of coil(D)÷wire diameter(d)
Waal correction coefficient(K)={(4×spring index)-1}÷{4×spring index-4}+(0.615÷spring index)
stress of the spring(T)=(8×Waal correction coefficient×mean diameter)÷(Pi×wire diameter to the 3)×load
spring index ⇒ 15.0÷2=7.5
Waal correction coefficient ⇒ {(4×7.5-1)/(4×7.5-4)}+(0.615÷7.5)=1.1974
stress of the spring ⇒ {(8×1.1974×15.0)÷(3.14×2.0^3)}×46.52=266.097N/㎟
